Skip to main content

What is Local Attraction in Surveying? - Civil Engineering

Surveying involves the measurement of lot of readings. Local attraction is one of the important concept of surveying. Bearings of lines are measured and they depends on magnetic meridian which gets affected by magnetic forces at site. So, calculations need to be done separately to rectify the readings of bearings.

Without a further delay, let's how all these calculations can be done properly so as obtained correct readings of bearings of line.

Local attraction in surveying

What is Local Attraction?

  • A magnetic meridian at a place is established by a magnetic needle which is uninfluenced by other attracting forces.
  • Some of the sources of this effect are: magnetite in the ground, wire carrying electric current, steel structures, railroad rails, underground iron pipes, keys, steel-bowed spectacles, metal buttons, axes, chains, steel tapes etc.​
  • The local attraction at a particular place can be detected by observing the fore and back bearings of each line and finding its difference.​
  • If Difference between Fore and Back bearing = 180 degrees for a station then, that station is free from Local attraction.

Elimination of Local Attraction:

  • First check whether any one of the station is free from local attraction or not. This can be checked from difference of fore bearing and back bearing.
  • In case there are such stations, use their bearing values and find others’ values.
  • If there are no such stations free from local attraction, then, included angles will be taken into calculation to rectify all bearings values.

There are two types of questions in this topic and we have discussed here one-one from both types. Make sure to watch it carefully for better understanding.

Type(1)- There are stations free from Local Attraction

(Q) The following bearings were observed while traversing with a compass. Determine the corrected Bearings of lines.

There are stations free from Local Attraction

Solution:

  • Observed back bearing of CD (i e 209°10’ is correct as station D is free from local attraction). 
  • Therefore, the correct fore bearing of CD will be 209°10’ - 180° = 29°10’, while the observed bearing is 29°45’. 
  • The correction required at C equals to -35’.
  • As in case of line BC, back bearing at C = 277°5’ - 35’ = 276°30’ and fore bearing = 276°30’ - 180° = 96°30’. Therefore, correction required for station B = -15’.
  • Similarly, do it for station A.

Type(2) - No such stations are free from Local Attraction

(Q) The following bearings were observed in running a closed traverse. Determine the correct magnetic bearings of the lines.

No such stations are free from Local Attraction

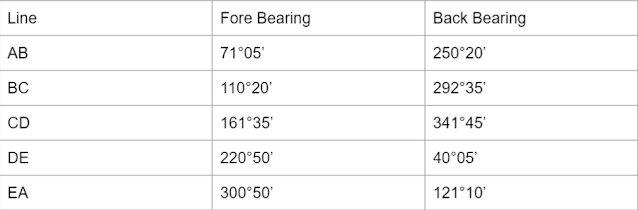
This can be solved using include angles. Try this on your own now, I will upload the solution and will mention it here.

Labels:

Comments

Post a Comment

Feel free to contact us.

Popular posts from this blog

Kunduz Tutor Registration, app, earnings - All you need to know

Kunduz is becoming a leading platform for asking doubts for JEE Mains and NEET exams and get cleared your doubts in a very less time.   About Kunduz Tutor app Probably, you may have listened about different apps/websites where you can work as a tutor and also make part-time income by clearing doubts.   Kunduz is totally safe to use . Some of such famous websites are like Toppr, Chegg, Kunduz , etc.  Toppr is also another website on which one can work as a tutor. This is our second tutorial to earn money by solving JEE / NEET questions . Today we will discuss briefly about Kunduz app and some below points: How can you work as a Tutor on Kunduz? Who can apply for Kunduz Tutor? How are payments given to tutors?  What is the registration process of Kunduz tutor? Payment proof. Is it worth? How can you work as a Tutor on Kunduz? On Kunduz it is something like 1-on-1 tutoring. First of all, during registration process you can select any one subject from Mathemati...
6 comments
Read more

Apps to solve JEE questions and earn 100$/month

H ello friends, welcome to our website News on Crunch - Informative News . Generally, after JEE when we go to college we want to have some amount of money for our personal expenses and often we want to earn it on our own.  So, we will talk about some of the great apps to solve JEE questions and earn $100/month depending on your work and the time you devote to those apps. I have tried many apps but the two apps which I found most helpful were Toppr and Kunduz tutor app. So, Let’s get started to know about the whole registration, earning and withdrawing process in these apps. Toppr App So in this app you have to solve the doubt of jee aspirants. Depending on the number of doubts you can earn money.  To start with this app first of all you have to get the missing certificates and suppose, if you're trying for Mathematics then you have to get free certificates general aptitude, logical reasoning and Mathematics. Those questions are generally of intermediate level.  You c...
2 comments
Read more

Is Kunduz app safe? Kunduz tutors salary and earnings

Kunduz is one of the best platforms to get your doubts solved of JEE and NEET exams. They have two platforms one for students and other one for tutors. Tutors have chance to solve questions posted by students and earn money. Kunduz tutor app is a platform where you can solve questions and earn money . And a different app name ' Doubt Solving app, Maths, JEE Mains, NEET - Kunduz ' is also available where students can post questions. For posting questions, students need to have subscription. Is Kunduz app safe? Kunduz app is 100% safe both for students and tutors. It is established brand in digital education which provides personalized solution of students' doubt in 30 minutes. For students: If you are JEE or NEET aspirants, then you can take their plans so as to ask up to unlimited questions. There are also other platforms like Doubtnut , Gradeup, Toppr, etc. So, ask your friends and choose the one which best suits you. They also have huge questi...
5 comments
Read more